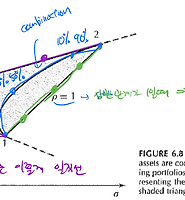
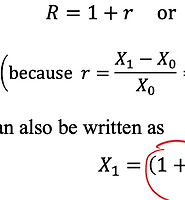incastle의 콩나물
Chapter4. Term Structure of Interest Rate || Part.3 본문
Short Rates
- short rates : a simpler way to calculate all future spot rates
모든 걸 step by step으로 계산하는 건 너무 귀찮아.....한 번에 계산하는 방법임 - Short rate at time k is the forward rate from k to k+1
k에서 플러스 1만 함(무조건 1년이네)
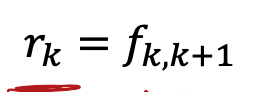
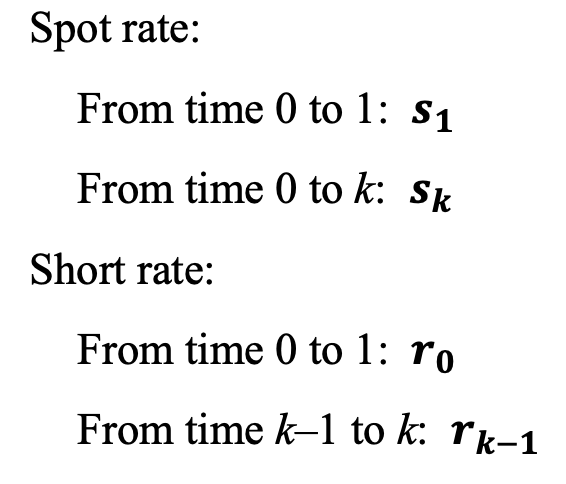
- spot => 끝나는 점 표기 / forward rate => 시작 + 끝나는 점 표기 / r(short) => 시작일만 표기
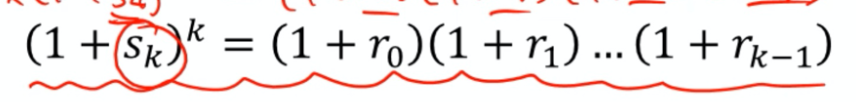
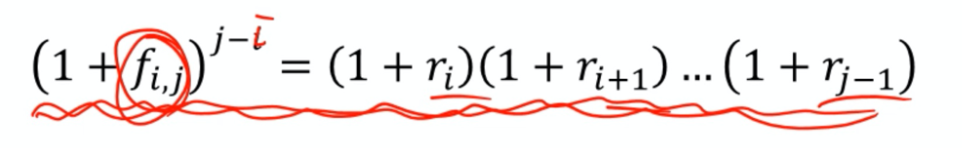
- Short rates form a convenient basis for generating all other rates
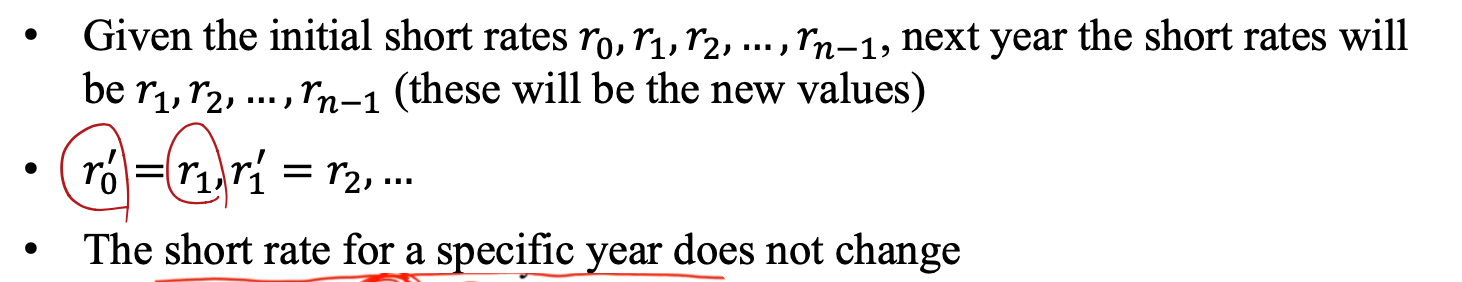
4.8 Duration
Duration
-
duration is a measure of interest rate sensitivity
-
In Chapter 3, duration was the sensitivity with respect to yield
-
In the term structure framework, yield is not a fundamental quantity
기간에 따라서 yield는 바꼈었어서 fundamental이 아님 -
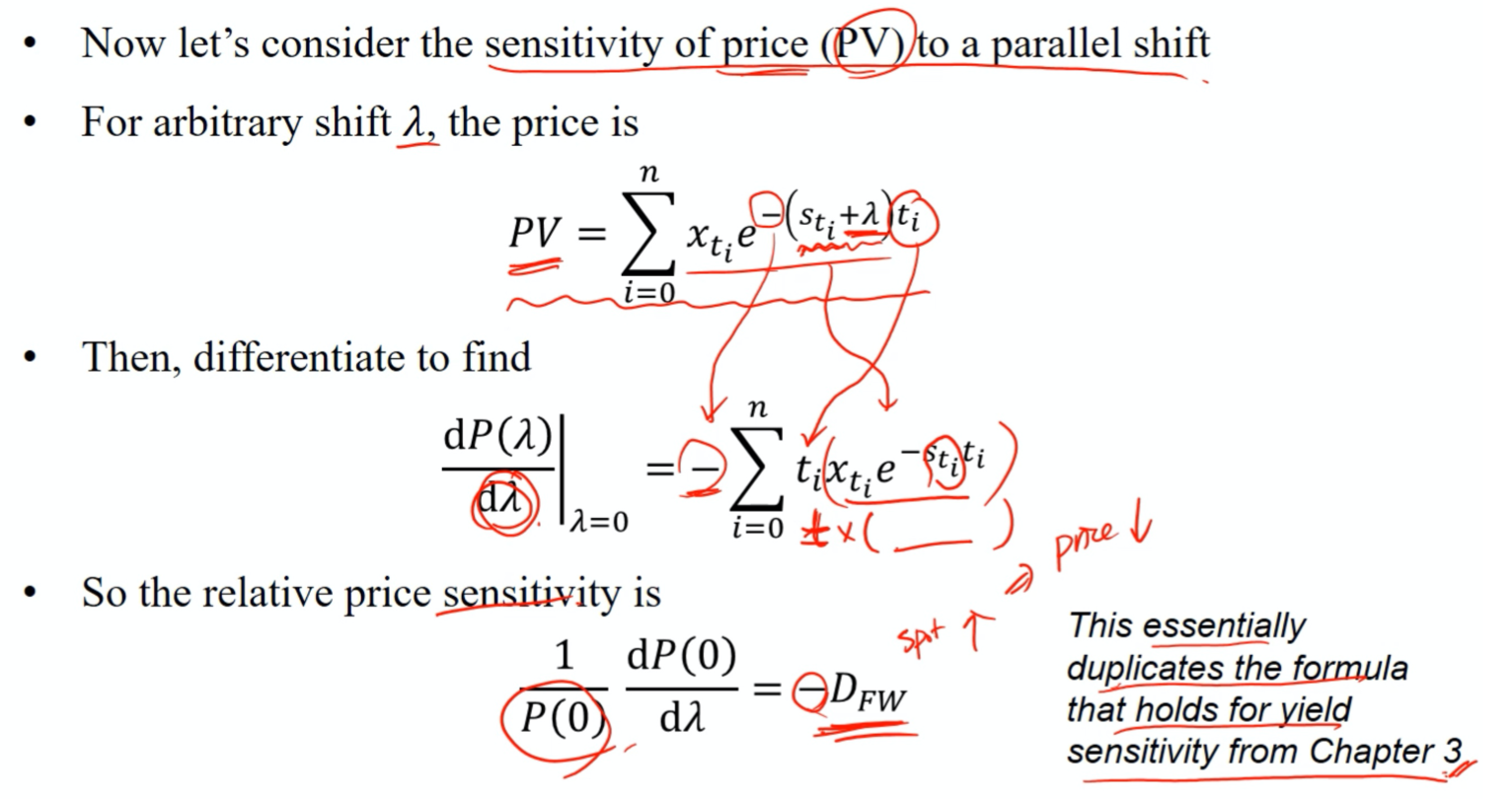
Instead, we can consider parallel shifts in the spot rate curve
그래서 spot rate curve를 활용하는 것
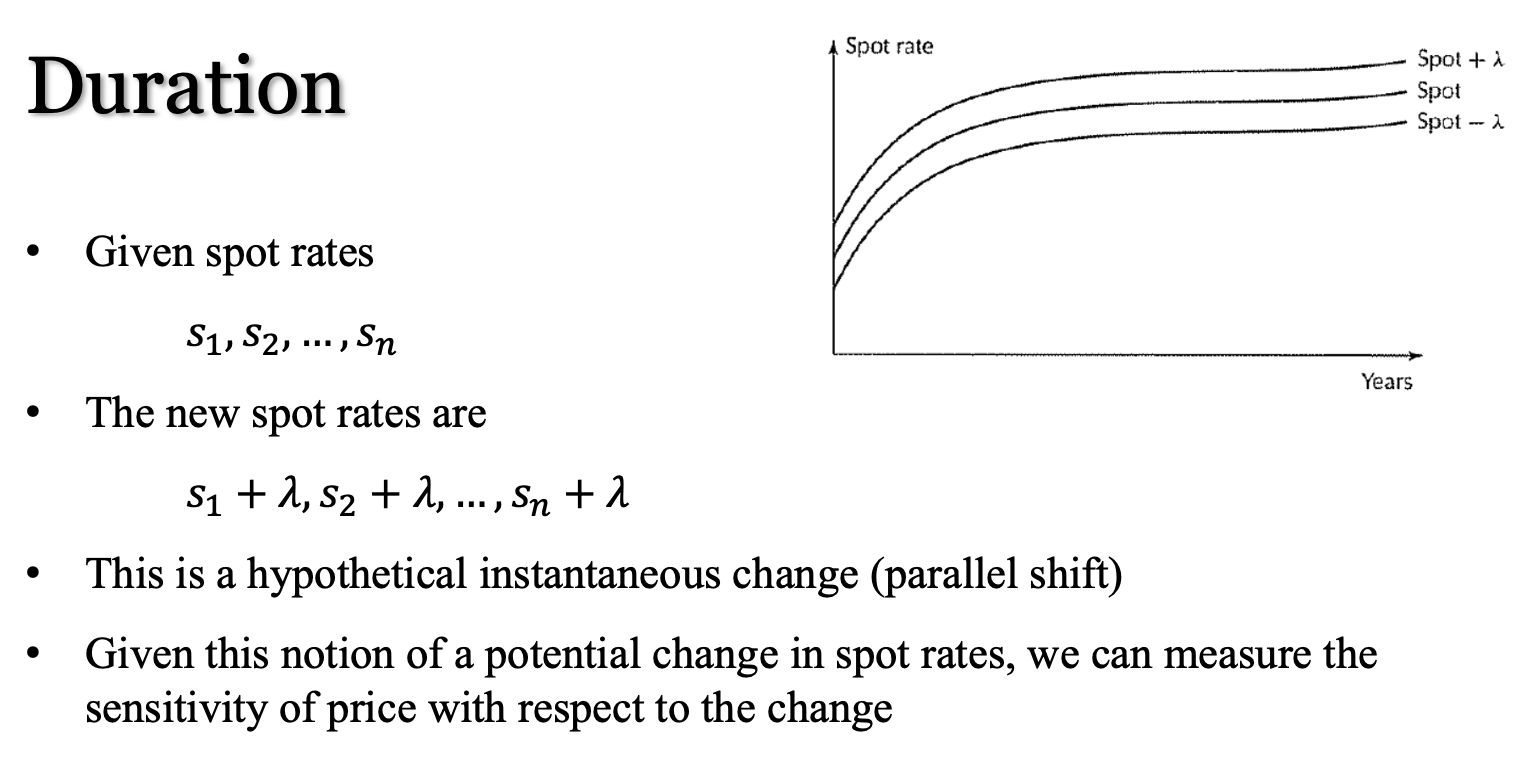
Fisher-Weil Duration
- Best expressed using continuous compounding
- Given a cash flow series (X_t0, X_t1, X_t2, .... X_tn) and spot rate curve s_t, the present value is

- Then, the Fisher-Weil duration is
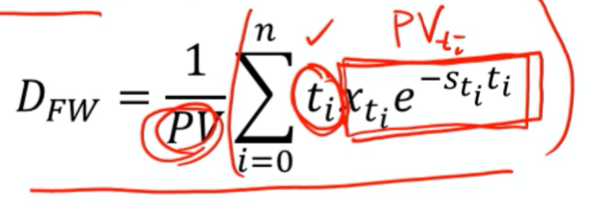
- Note that this corresponds to the definition of duration as a PV-weighted average of the cash flow times
Summary of Chapter 4
-
Yield curve
-
Term structure
-
Spot rates and corresponding discount factors •
-
-
Forward rates
-
Implied forward rate calculation
-
-
Expectation dynamics
-
Spot rate forecasts and short rates
-
-
Duration
'20-1 대학 수업 > 금융공학' 카테고리의 다른 글
| Chapter 6. Mean-Variance Portfolio Theory || Part 3 (0) | 2020.05.17 |
|---|---|
| Chapter 6. Mean-Variance Portfolio Theory || Part 1 (0) | 2020.05.16 |
| Chapter4. Term Structure of Interest Rate || Part.2 (0) | 2020.04.20 |
| Chapter4. Term Structure of Interest Rate || Part.1 (0) | 2020.04.17 |
| Chapter3. Fixed-Income Securities || Part2 (0) | 2020.04.16 |
Comments